Dewey and Cycles
Edward R Dewey (1895-1978) formed the Foundation for the Study
of Cycles in 1940.

This is a selection of a small part of his work and that of others that
he collected.
The works of Dewey are available from the Foundation for the Study
of Cycles in the form of the
"Cycles Classic Library Collection" which in its four volumes contains
the wisdom of 40 years cycle research.
Now a new organisation, the Cycles research Institute, has begun to
continue the work of Dewey and the Foundation for the Study of Cycles.
Cycles Research Institute
Foundation for the Study of Cycles
What are Cycles?
by Edward R Dewey, 1951
Cycles are the simplest thing in the world, at least in principle. Let
me give you an example.
Suppose you are visiting my house and, looking out of the window,
notice a bus pass by at 10:00 a.m. Half an hour later at 10:30 you notice
another bus pass. At 11:00 you see another one, " Ah ha! " you say. "Buses
here run every thirty minutes." You have discovered a cycle all by your
little lonesome, without benefit of slide rule or gobbledegook. Cycles
are just as simple as that.
Now what?
Well, first of all, you have a basis for predicting the probabilities
of the future. If we go in to lunch and come out of the dining room at
1:05 you will know that you probably missed the 1:00 o'clock bus and that,
if the cycle is continuing, your next bus will pass in 25 minutes. So you-chat
for about 20 minutes and leave at 1:25 so as to have to stand in the wind
and the rain the least amount of time.
Of course the schedule may have changed, or the bus have been
delayed by an accident. You can't count for sure on a bus at 1:30. You
are merely playing probabilities.
Regularity Gives Predictability
Where you have regularity you have predictability-at least to the extent
that the regularity governs, and is not present by chance.
Now let's do some more supposing.
You are overlooking a street near the center of a small town.
Every ten minutes or so 10 or 15 people come along, more or less in a bunch.
Another cycle! Again you can predict (with qualifications). More than this,
with this regular result appearing before you, you have a right to assume
a cause - if the time intervals have been regular enough and have repeated
enough times so that the behavior cannot reasonably be the result of chance.
You don't know the cause but there is no law against guessing.
So you guess that, there is a bus station around the corner and that every
ten minutes a bus comes in and discharges its passengers. If you then find
out that there is a bus station, and that a bus does come in every ten
minutes, your guess is bolstered. It is bolstered still more if you find
that the buses arrive just about the time your bunches of people have been
coming. But you still don't know that your people are coming from the buses.
You would have to go out on the street and go around the corner to find
out for sure.
Other Cycles
You continue to watch and count the people. You see other patterns. Every
other ten-minute group of people is bigger. Perhaps there is a second bus
with a twenty-minute schedule that reinforces the crowd from the first
bus.
This is really the whole story about cycles.
You can predict that part of the traffic that comes in regularly
recurring bunches. You can not predict by your 10- or 20-minute cycles
the crowd of people that will come along when the feature of the local
movie house is over. Your predictions are only partial. But they are good
as far as they go if the cycles keep on coming true, and if you have timed
them right. Moreover, by finding something else (in the example given,
a bus schedule) with regularly recurring cycles of the same length you
get a clue as to possible cause and effect and relationship - But to make
sure, you need to run your clue down. Where you have partial regularity
you have partial predictability. For example, suppose you have a chart
recording your heart beats over the period of a minute. Now look at the
first half of it only. If you count 30 beats in the 30 seconds You have
under examination you will not be far wrong if you forecast another beat
at 31 seconds, another at 32 seconds, etc.
Perhaps, if your heart speeded up a little, you would be quite
wrong if you made your projection ten beats ahead, but by making your forecast
only a beat at a time and revising your forecast each beat, you could probably
make your prediction come out fairly well.
Another example of partial predictability: Suppose you knew that
February 1st is, in New York City, on the average, the coldest day of the
year. Suppose, on the basis of this information, you forecast February
1, 1952 as the coldest day of next winter. It is almost certainly true
that February 1st will be colder than August 1st, but in any given year
it may not be the very coldest day by as much as one or two months. The
cycle of the year influences the weather but it does not completely dominate
it. From a knowledge of the cycle of the year alone you could forecast
the coldest day only with wide tolerances, yet the cycle of the year is
very real.
Almost everything fluctuates with rhythm - that is, in more or
less regular cycles. Putting it another way, almost everything acts as
if it were influenced by regularly alternating up and down forces which
first speed it up and then slow it down.
Random Ups and Downs Too
Everybody would know this fact were it not for two things: First, in addition
to the cycles there are accidental (random) fluctuations in things, too.
These randoms hide the regularities so that at first glance you do not
see them. Second, things act as if they were influenced simultaneously
by several different rhythmic forces, the composite effect of which is
not regular at all.
If we had several moons, the ups and. downs of the tides would
be very irregular. All the other moons would mix things up. It might have
taken us much longer to find out about the tides.
Separating Cycles Easy
If you have a long enough series of figures with which to work it is not
too hard to separate the different regular cycles from each other. When
this has been done, you can project each regular cycle into the future
- Then you can easily find out the combined or composite future effect
of all the various cycles. When you have done this you have a preview of
what is going to happen (a) if the cycles continue, and (b) except as the
cycles may be upset or distorted by accidental random non-cyclic events.
"Why wouldn't the cycles continue?" you may ask.
I'll give you one reason: The cycles may have been present in
the figures you have been studying merely by chance. The ups and downs
you have noticed which come at more or less regular time intervals may
have just happened to come that way. The regularity - the cycle - is there
all right, but in such circumstances it has no significance. If you are
zealous enough you can find regularity in almost anything, including random
numbers where you know that the regularity has no significance and know
it will not continue.
Many Repetitions Needed
How can you tell in any given instance whether or not the regularity you
see is the result of a real underlying cyclic force which will continue
to fluctuate regularly in the future?
The answer is, if the cycle has repeated enough times with enough
regularity and with enough dominance, the chances are that it is the result
of real cyclic forces.
Let me give you an example: Pick up a pack of playing cards and
start to deal. The first card is red, the second is black, the third is
red, the fourth is black. You have two waves of a regular cycle, red, black;
red, black. But this sequence could easily come about by chance. You continue
to deal: red, black; red, black. Four times in a row now, this regular
alternation. It could still be chance, but it couldn't be chance very often.
Continue to deal. Red , black;,red, black; red, black. Seven times now!
It could still be chance, but it is less and less likely. It begins to
look as if somebody had stacked the cards. You go through the entire deck.
Twenty-six times! "Somebody certainly stacked the deck," you say."It
couldn't happen this way by chance once in a million."
Less and Less Likelihood of Chance
Exactly the same sort of reasoning applies to the cycles you see in the
ups and downs of the stock market, or the sales of your own company, or
the weather, or anything else in which you may be interested. The more
the cycle has dominated, the more regular it is, and the more times it
has repeated, the more likely it is to be the result of a real cyclic force
that will continue. If it has not dominated enough, or has not been regular
enough, you must have more repetitions to get equal assurance.
Well then, supposing that there are these rhythmic cycles in something.
Suppose further that you have some knowledge of cycles. So what?
By using nothing more complicated than simple arithmetic you can
find the cycles. By seeing how many times each cycle has repeated in the
past you can have a pretty fair idea of its significance (i.e. whether
or not it will continue). And by projecting all the significant cycles
into the future you can get some light on what is ahead insofar as the
cycles govern.
Cycle Forecast Like a Weather Forecast
In weather we are used to forecasts in terms of probabilities. "The probability
for the Pittsburgh area is for snow." When you hear such a forecast on
the radio you don't rush out to put on your chains. The weather man may
be wrong. You wait for the snow to fall. Then you put on your chains. But
the forecast warns you that snow is likely, and on the strength of this
fact you do make sure that you have your chains with you. If you are to
make use of cycles in your business or your stock market forecasting you
are going to have to use the same approach. Moreover, just as you refrain
from shooting the weather man when he is wrong, I hope you will refrain
from shooting the cycle analyst too.
Cycles Indispensable
Cycles are not the whole answer, but on the other hand they are indispensable
in attempting to arrive at the whole answer.
Cycles remind me of women. Women are not perfect (with individual
exceptions, I hasten to add). But they are the best thing so far invented
for the purpose. Until something better comes along we will have to make
shift with them as they are - or else miss the values they have to offer.
Let's find out all we can about them!
Cycles Show Us Our Ignorance
The second reason that the study of cycles is important arises from the
fact that wherever you have rhythmic variation - or for that matter pattern
of any sort - you probably have a cause. For example, if you scatter iron
filings on my desk you would expect them to be distributed at random over
its surface. If, to the contrary, you find them arranging themselves into
a pattern, you have a right to assume that some unknown force is present
- perhaps a powerful magnet in my top drawer.
Similarly, if you find pattern, or more specifically rhythm (reasonably
regular cycles), in the alternate thickness and thinness of tree rings
or rock strata, or in the abundance of insects, or in the prices of common
stocks, you may be sure that there is a cause for such behavior. Also you
may be sure that if you do not know that cause you do not fully understand
the behavior with which you have to deal. Thus the study of cycles reveals
to us our ignorance, and is therefore very disturbing to people whose ideas
are crystalized. "If there are regularly recurring ups and downs in business
or in prices, all I have ever learned is wrong" an eminent economist once
told me; and he added, in a moment of unusual candor, "I simply cannot
afford to accept such an idea. All my life's work would be ruined." Many
doctors in the days of Pasteur must have felt much the same way about germs.
Identical Cycles Suggest Interrelationship
The study of cycles has a third value. Such study can indicate possible
cause-and-effect relationships, thus helping to solve the very problems
it reveals.
For example, when I was a boy I noticed that the moon came up
at about an hour later each night. When I visited the seashore I noticed
also that the tide came in half an hour or so later each twelve hours.
I did not have the wit to go on from there to note that two consecutive
tides were delayed by exactly the same amount of time as was the moon,
and therefore that there was perhaps some connection between the two. I
did not have the wit to make this observation, I say, but one of the older
astronomers did. In doing so he demonstrated the third value of a knowledge
of cycles: Where the rhythms in two different phenomena have the same average
time span, you are justified in suspecting a possible direct or indirect
interrelationship between the phenomena.
Scientists Interested
For these three reasons many scientists are interested in the study of
cycles.
First, it is the business of science to predict; second, it is
the business of science to solve mysteries and to learn the "how" of things;
and third, the true scientist welcomes any tool that gives him hints as
to possible cause and effect relationships. Perhaps 3,000 scientists, the
world over, have concerned themselves with the subject, and have written
scientific papers embodying the results of their observations.
Scientists however, like all the rest of us, are pretty self centered.
Thus the mammalogist interested in cycles in animal abundance is usually
not much interested in geological cycles,or in astronomical cycles, or
in business cycles. He is primarily, and usually exclusively, a mammalogist.
Likewise the men in each of perhaps thirty different aspects of natural
and social science are likewise specialists, each involved in his own little
field.
However, the techniques of cycle analysis are the same whether
one is studying biological data or financial data. And - much more important
- it is only by studying cycles in all sorts of phenomena, and finding
which phenomena have cycles of identical average time span, that we are
likely to get hints of cause and effect relationships.
A New Science
These two facts cry aloud for the creation of a new science - the science
of cycles - which is concerned with rhythmic fluctuation per se,
which will develop techniques of cycle analysis, which will isolate cycles
in all the 30 or 40 different branches of science where cycles are important,
and which, having assembled enough facts, will perhaps someday venture
to advance some theories in regard to cause and effect.
The Foundation for the Study of Cycles was created, in 1940, to
found such a new science, and to develop it to the point where it could
serve mankind. In the eleven years which have elapsed since its creation,
the Foundation has made slow but steady progress.
It should be clear from the above remarks that the Foundation
is purely an educational and scientific body and in no sense a commercial
organization. It exists, not to make money but to serve mankind.
An eminent group of scientists and administrators have lent their
names to the Committee of the Foundation. Various scientific societies
have appointed advisors to help with its awards, and many individual scientists
have joined the Foundation as scientific members. On its part, the Foundation
has tried to maintain the high scientific standards of the many universities
and institutions here and abroad which are, through their professors, connected
with it, and I think we have pretty well succeeded.
Cycles Can Help You, Too
The research of the Foundation is of immediate practical value to the average
citizen, too. By uncovering cycles in production and trade it throws light
on the probabilities of booms and depressions. By uncovering cycles in
international conflict it throws light on the probabilities of war. By
uncovering cycles in the prices of commodities and of securities it throws
light on the probabilities of panics and of other financial disturbances.
The results of the Foundation's research are made available to
the general public by means of reports, bound together and issued 12 times
a year in - the form of a magazine called Cycles - A Monthly Report.
Cycles Graphs
The following graphs come from Dewey's articles in Cycles magazine and
later published in the above mentioned collection. Because they are historical
data they do not include the recent past. Please excuse the quality of
the reproduction. Note also that for most of the graphs Dewey removed the
trend of the series by subtracting a moving average from the data with
a length approximately equal to the cycle period. The dotted lines show
an "ideal" regular cycle of the stated length.
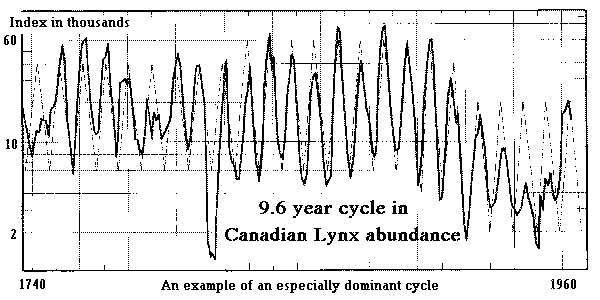
Canadian Lynx abundance has varied by a very wide factor on a 9.6 year
cycle. This is very clear cycle with over 230 years data and is considered
to be one of the clearest cycles known.

The 9.6 year cycle has been found in the populations of insects, fish and
mammals. It is also present in the propensity for humans to have heart
attacks. This seems entirely weird until the last member of the group is
added, the variation in ozone. Is this a hint at the connection?
The following two graphs are presented as percentage variations from
a trend.
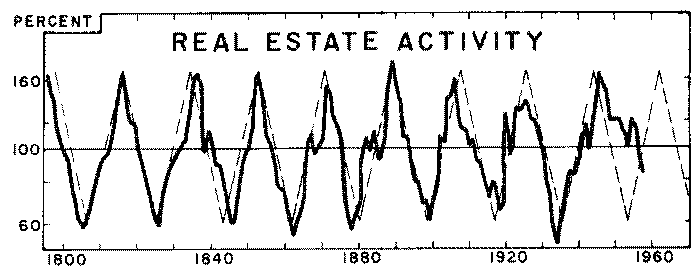 Real estate activity is another clear cycle, this time of about 18 years.
Real estate activity is another clear cycle, this time of about 18 years.

Wholesale prices in different continents show not only the same 9 year
period, but also are in phase. This tendency for equal periods, even for
unrelated series, to have synchronised phases is called "Cycles Synchrony"
by Dewey.
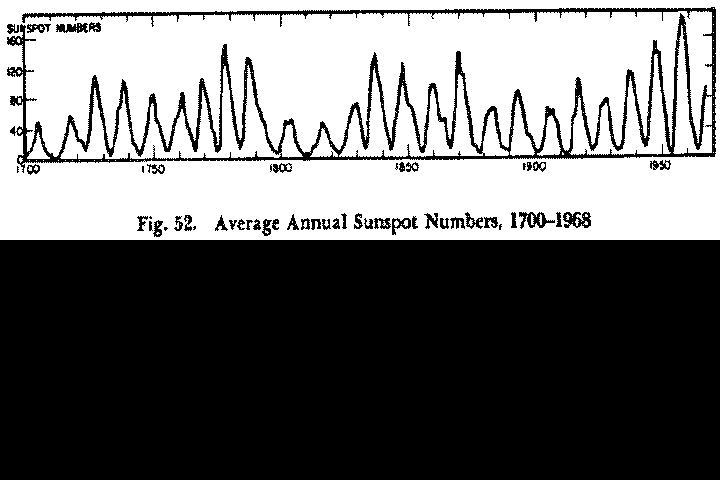
The 11 year Sunspot Cycle is one of the better known cycles.
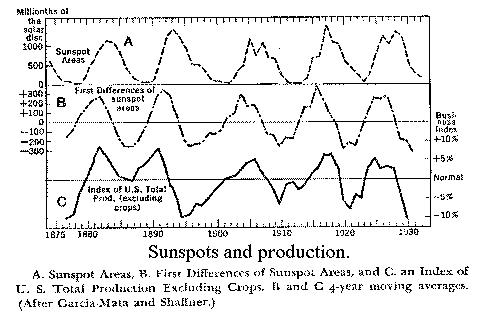
Industrial production is shown to be closely correlated with the rate of
change of sunspot activity. This is an area which has often been criticised,
but the graph is interesting. It would be interesting to have an update
on this old graph to see what has happened since.
Return to Ray Tomes index page
Cycles Research Institute






